Harder 3U - Inequalities (1 Viewer)

[RivalryofTroll]

If a, b, c, d > 0, show that:

a) (a/b) + (b/a) ≥ 2

My solution:
(a-b)^2 ≥ 0
a^2 + b^2 ≥ 2ab
(a^2 + b^2)/ab ≥ 2
Therefore, a/b + b/a ≥ 2

How do you use part (a) to do parts (b) and/or (c)

b) (a/b) + (b/c) + (c/a) ≥ 3

c) (a/b) + (b/c) + (c/d) + (d/a) ≥ 4

 

[Sy123]

I'll give you the first half of it, and let you finish it off.

For b):

It seems we need to somehow connect, a/b to b/a, b/c to c/b and c/a to a/c, so that we can get some 'cancellation' and get a definitive number.
One may then proceed to add something to both sides but this doesn't look like it will lead anywhere.
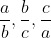
Now notice that if you multiply any 2 of the term, we will get one of these:

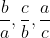
i.e. if you multiply the first 2 terms in the inequality that we need

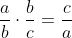
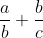
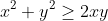
This is important since it will allow us to establish the connection, so how do we go from a sum: to a product? We can use the basic inequality: , we need an addition of 3 terms however, so lets add 3 of htose inequalities side by side to yield the familiar inequality:



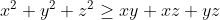
Substituting appropriate values in:



We don't really want to deal with the squares of a/b b/c and c/a, so lets force to to become just a/b b/c and c/a



I'll let you continue on from here however, if you've tried and can't get it I'll finish it off for you

======

c) Unfortunately, if we do something similar here to above, we will have to resort to using the inequality:



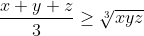
Which I don't want to do, nor do I think the HSC will accept it unless you prove it, if I were allowed to use this inequality then the first one becomes trivial, by just subbing in x=a/b, y=b/c, z=c/d

I'll post again if I can find another solution to this one.

EDIT: Please ignore completely, its pretty wrong =)

 

[HeroicPandas]

Finally after some death

a) (a/b) + (b/a) ≥ 2 .........[1]

c) (a/b) + (b/c) + (c/d) + (d/a) ≥ 4

Same method as (b), but this time its easier, im gonna use AM-GM inequality on each pair of colours (red and blue)

First pair: a/b and b/c


x-->a/b and y--> b/c


Second pair:
Similarly, x--> c/d, y -->d/a


Add both these,



note 1:

 

[obliviousninja]

Finally after some death

b) (a/b) + (b/c) + (c/a) ≥ 3
From [1]

now a -->c,

now from [1] b-->c,

Add all these 3 equations, and the answer appears immediately

This is probs a stupid question, but at school we haven't covered inequalities yet, but how does the answer immediately?

 

[braintic]

b) (a/b) + (b/c) + (c/a) ≥ 3
From [1]

now a -->c,

now from [1] b-->c,

Add all these 3 equations, and the answer appears immediately

I must be missing something there. The only way the answer comes out immediately is if a/b + b/c + c/a = b/a + c/b+ a/c, and I don't believe that is the case.

 

[HeroicPandas]

This is probs a stupid question, but at school we haven't covered inequalities yet, but how does the answer immediately?

it doesnt lol, made a mistake

I must be missing something there. The only way the answer comes out immediately is if a/b + b/c + c/a = b/a + c/b+ a/c, and I don't believe that is the case.

yes i made a mistake haha

 

[obliviousninja]

it doesnt lol, made a mistake

yes i made a mistake haha

Can you put out the correct solution if possible.

 

[braintic]

If you are permitted to use the general AM-GM inequality, it comes out easily.

 

[seanieg89]

Proving the n=3,4 AM-GM is almost immediate from the n=2 AM-GM.

As I am quite sure you would not be allowed to assume AM-GM so I would just do the above. (unless the question explicitly says "hence").

Any other proof is just AM-GM in disguise.

 

[HeroicPandas]

Can you put out the correct solution if possible.

look at the Sy's post above, he made a great solution

 

[Sy123]

I tried to look at my notes where I 'solved' this question since I don't remember it, it turns out that in my 1am stupor I made a mistake in squaring (a/b + b/c + c/a)^2. Which led me to a cool 'solution' which had a substitution.
But yeah its wrong lol, sorry about that.....