-
Looking for HSC notes and resources? Check out our Notes & Resources page
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
HSC 2016 MX2 Integration Marathon (archive) (1 Viewer)
- Thread starter leehuan
- Start date
- Status
- Not open for further replies.
Drsoccerball
Well-Known Member
- Joined
- May 28, 2014
- Messages
- 3,647
- Gender
- Undisclosed
- HSC
- 2015
Re: MX2 2016 Integration Marathon
Can easily be solved by a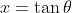 substitution.
substitution.
As a wise man named DJ Khaled once said
Another one:
 \cdot \cos{x}}}} dx )
Can easily be solved by a
As a wise man named DJ Khaled once said
Another one:
Drsoccerball
Well-Known Member
- Joined
- May 28, 2014
- Messages
- 3,647
- Gender
- Undisclosed
- HSC
- 2015
Re: MX2 2016 Integration Marathon
 ? Let me have a proper attempt.
? Let me have a proper attempt.
The one I said was 0Okay, would people like me to post a solution to my old one then? Or is anyone still trying it?
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 2016 Integration Marathon
 .
.
Will post my solution later if it remains unsolved here.
By all means, I think it's fairly funThe one I said was 0? Let me have a proper attempt.
Will post my solution later if it remains unsolved here.
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 2016 Integration Marathon
Okay, I think it's been long enough so I will post my solution.
If you feel you are making progress then keep trying yourself before reading the below!!
For starters, let's assume a and b are positive, as changing the sign of one of these guys literally just changes the sign of the integral, as sine is odd.
\sin(bx)}{x^2}\, dx\\ \\ = \lim_{R\rightarrow \infty}\int_{-R}^R \frac{\cos(a-b)x -\cos(a+b)x}{2x^2}\, dx \\ \\ = \lim_{R\rightarrow \infty}\int_{-R}^R \frac{\sin^2((a+b)x/2)-\sin^2((a-b)x/2)}{x^2}\, dx)
by basic trigonometric manipulations (specifically, product to differences and the half angle formula.) The motivation for the second manipulation is that the denominator tends to zero as x -> 0. This means that if we want to split the integral up as a sum or difference, the parts of the numerator should each tend to zero as well. so sines are good but cos's are bad.
So we have now reduced it to studying integrals of the form
=\lim_{R\rightarrow \infty}\int_{-R}^R \frac{\sin^2(cx)}{x^2}\, dx\\ \\ =c\lim_{R\rightarrow \infty}\int_{-cR}^{cR} \frac{\sin^2(x)}{x^2}\, dx \\ \\ =c\lim_{R\rightarrow \infty}\int_{-cR}^{cR}\sin^2(x)\frac{d}{dx}(-1/x)\, dx\\ \\ =c\lim_{R\rightarrow \infty} \int_{-cR}^{cR} \frac{\sin(2x)}{x}\, dx\\ \\ = c\lim_{R\rightarrow \infty}\int_{-2cR}^{2cR}\frac{\sin(x)}{x}\, dx )
where the third inequality came from integrating by parts, noting that the boundary term decays as 1/R and hence vanishes in the limit.
Now it is tempting to conclude that this final quantity is nothing more than the principal value integral of sin(x)/x over the real line. HOWEVER, there is a subtlety caused by c's sign! Note that if c is negative, then this final integral is negatively oriented! This means that in this case we will actually get the negative of the principal value integral. Hence we have
=\textrm{sgn(c)}\cdot c\pi)
where the sgn function is defined to be 1 on the positive reals, -1 on the negative reals, and 0 at zero.
Plugging this back into our most recent expression for I, we get
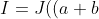/2)-J((a-b)/2)=\frac{(a+b)\pi}{2}-\frac{(a-b)\pi}{2}\textrm{sgn}(a-b)\\ \\=\frac{(a+b)\pi}{2}-\frac{(\max(a,b)-\min(a,b))\pi}{2}\\ \\=\pi\cdot\min(a,b).)
The surprising upshot: the integral does not vary if you change the larger of the two parameters, but it does if you change the smaller one!
Okay, I think it's been long enough so I will post my solution.
If you feel you are making progress then keep trying yourself before reading the below!!
For starters, let's assume a and b are positive, as changing the sign of one of these guys literally just changes the sign of the integral, as sine is odd.
by basic trigonometric manipulations (specifically, product to differences and the half angle formula.) The motivation for the second manipulation is that the denominator tends to zero as x -> 0. This means that if we want to split the integral up as a sum or difference, the parts of the numerator should each tend to zero as well. so sines are good but cos's are bad.
So we have now reduced it to studying integrals of the form
where the third inequality came from integrating by parts, noting that the boundary term decays as 1/R and hence vanishes in the limit.
Now it is tempting to conclude that this final quantity is nothing more than the principal value integral of sin(x)/x over the real line. HOWEVER, there is a subtlety caused by c's sign! Note that if c is negative, then this final integral is negatively oriented! This means that in this case we will actually get the negative of the principal value integral. Hence we have
where the sgn function is defined to be 1 on the positive reals, -1 on the negative reals, and 0 at zero.
Plugging this back into our most recent expression for I, we get
The surprising upshot: the integral does not vary if you change the larger of the two parameters, but it does if you change the smaller one!
Last edited:
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 2016 Integration Marathon
Note that following my own suggestion, I assumed knowledge of the sin(x)/x integral in order to compute the above.
So treating it as a separate question, prove that:
}{x}\, dx=\pi)
I will give students a little more time to have a crack at this famous integral before I post an evaluation of it too.
Note that following my own suggestion, I assumed knowledge of the sin(x)/x integral in order to compute the above.
So treating it as a separate question, prove that:
I will give students a little more time to have a crack at this famous integral before I post an evaluation of it too.
Re: MX2 2016 Integration Marathon

Can easily be solved by asubstitution.
Re: MX2 2016 Integration Marathon
 \cdot \cos x} &= \int \frac{2 \sec x}{\sin x - \sqrt{3} \cos x} \, dx = 2 \int \frac{\sec^2 x}{\tan x - \sqrt{3}} \, dx\end{align*})
 \cdot \cos x} &= 2 \int \frac{du}{u - \sqrt{3}}\\&= 2 \ln |u - \sqrt{3}| + \cal{C}\\&= 2 \ln|\tan x - \sqrt{3}| + \cal{C}\end{align*})


As a wise man named DJ Khaled once said
Another one:
 \cdot \cos{x}}}} dx )
Paradoxica
-insert title here-
Last edited:
Paradoxica
-insert title here-
Paradoxica
-insert title here-
Paradoxica
-insert title here-
Re: MX2 2016 Integration Marathon
An extension of the previous problem, I guess...
 Show that it is always possible to decompose any expression of the form$\\ \frac{p\sin^2x +q\cos^2x +r\sin x\cos x}{a\sin x+b\cos x} \forall \{a,b,p,q,r \} \in \mathbb{R} $ (A.K.A. use partial fractions)$ \\$ into a sum of 3 simpler expressions, subject to restrictions on the denominator.$ \\ $b) Suppose $a^2 +b^2 = c^2$. Evaluate the integral of the above expression.$)
An extension of the previous problem, I guess...
Last edited:
Drsoccerball
Well-Known Member
- Joined
- May 28, 2014
- Messages
- 3,647
- Gender
- Undisclosed
- HSC
- 2015
Re: MX2 2016 Integration Marathon
Do we actually have to watch out for the domain in integration ?
Do we actually have to watch out for the domain in integration ?
Re: MX2 2016 Integration Marathon
Yeah.Do we actually have to watch out for the domain in integration ?
Paradoxica
-insert title here-
Re: MX2 2016 Integration Marathon
Requires multi-valued gamma function with one real and one imaginary argument, according to Mathematica. So a reduction formula definitely exists.I haven't tried it yet.

- Status
- Not open for further replies.
