The Area Under a Parabola. (1 Viewer)

[U MAD BRO]

So I have this question saying show that the area enclosed by a parabola of the form 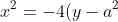and the x-axis is . (VOLUMES QUESTION)


I made y the subjects and integrated between 2a and 0 then multiplied by 2 since a parabola is symmetrical etc... and got .



But I was wondering whether you can do this with simpson's rule? I mean is there an alternative way other than integration to find the area under a parabola?

 

[lolcakes52]

Yes, because simpsons rule uses parabolic segments it should work out.... I think.

 

[U MAD BRO]

So simpson's rule gives you the exact area only if the curve is a parabola.

 

[asianese]

Yes. Simpson's rule is the approximation USING A parabola. Hence it will find the area under a parabola exactly.

 

[U MAD BRO]

Part (ii) of the question is the weirdest volumes question I've ever seen, it might be easy but I don't know how to do it =(
Please help me with it

FIND THE VOLUME OF THE SOLID

 

[asianese]

Does the question mean 'the two zeroes like on y=2x and x=5?? from the diagram the zeroes both lie on y=2x

 

[U MAD BRO]

Does the question mean 'the two zeroes like on y=2x and x=5?? from the diagram the zeroes both lie on y=2x

I think so.

The diagram is poorly drawn, should be x=5 and y=2x

 

[U MAD BRO]

BUMP!

 

[Carrotsticks]

I'll explain it to you at 5pm.

 

[U MAD BRO]

I'll explain it to you at 5pm.

oh ok, thank you, thank you, thank you a lot

 

[Johnstan]

carrot u must discriprine this user.

 

[U MAD BRO]

carrot u must discriprine this user.

why so many accounts siddy

 

[Johnstan]

why so many accounts siddy

only one brah.
who's alt r u haha?

 

[U MAD BRO]

only one brah.
who's alt r u haha?

This is my main =)
I'm new anyways.

 

[Johnstan]

This is my main =)
I'm new anyways.

oh ok hahah