Hard integral, help. (1 Viewer)

[5uMath]

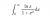
Can someone please help with the question above, but replace ln( x ) with ln^2 (x).

I am asked to prove it equals pi cubed on 8, but when I calculate residue, this is what I get up (maybe with negative) until i apply contour integration, to which the integral converges to 0.

Also, you must use cauchy residue theorem, cant be real analysis.

I dont know why I keep getting 0, I am following methods correctly and using formulae correctly...

 

[5uMath]

My guess is that you're getting zero because you assumed that

If you then apply the residue theorem using the 2 poles at , they end up cancelling out.

If that's the step you took, then I believe the mistake is in the very first line. You cannot simply halve the integral from to because . The integral needs to be split into 2 domains and evaluated separately. The video below shows how to integrate the expression in the image, but can be extended to the integral involving the by the same process.

It's been a while since I've touched this stuff, so I could be wrong.

I cannot believe I forgot to say this. Contour defined on epsilon <= |z| <= R, epsilon goes to 0 and R goes to infinity.

If you integrate over the full contour you would get 0 according to jordans lemma

 

[5uMath]

My guess is that you're getting zero because you assumed that

If you then apply the residue theorem using the 2 poles at , they end up cancelling out.

If that's the step you took, then I believe the mistake is in the very first line. You cannot simply halve the integral from to because . The integral needs to be split into 2 domains and evaluated separately. The video below shows how to integrate the expression in the image, but can be extended to the integral involving the by the same process.

It's been a while since I've touched this stuff, so I could be wrong.

I didnt halve. I integrated over the full contour defined, got the integral over the two semi circles =0, applied let z=-z then combined the integrals to get limits epsilon to R. In the process you get these other integrals, some which go to zero, and some which go to, collectively, pi cubed on 3 and then the 0 would appear

 

[5uMath]

The integrand is non-negative, so you can't get zero lol. Throw it into wolframalpha and you get 3.7578.

Thats exactly right. I know im wrong, but i dont want to result to calculators

 

[5uMath]

I cant see your latex text

 

[5uMath]

Oh right sorry, I misunderstood what you meant. I thought you realized that the integral must equal zero, rather than stating what you were doing. Either way, if you use the approach in the video, it should lead to the correct result.

The question is meant to be loge x squared. ive seen that and applied the same thing but get 0... ill send my answers tomorrow

 

[5uMath]

Yeh it took a while for it to load on my end, tex commands seem to be really slow on bos these days. I can see it now after I waited a bit and hit refresh a few times.

Yes I see it now.

I did split ln (-z) to ipi + lnz, and used it wherever it needed to be. That was the reason i kept getting 0

 

[5uMath]

Yeh might help if you detail your working. Either way it's been almost a long time since I've done this stuff so I don't know how much of it I can remember. Had to google the formula for the residue theorem was to refresh my memory.

Oh thats fine. I hope one day to take analysis after school. Not real analysis, never really into it, but i like complex and functional

 

[5uMath]

I have attached a copy of my solution.

Turns out i didnt get 0 this time, but got pi^3/4... do you see mistakes?

Thanks

 

[5uMath]

Your working out seems fine, not sure where the missing factor of a half is meant to be lol.

I honestly dont, but pi^3/8 is the value you got from wolfram yesterday, so definitely a mistake. But where......

 

[5uMath]

I found the mistake!!! Where i evaluated the integral containing (ipi + lnx)^2, i forgot to reconsider the second integral, I2, after proving I1 =-pi^3/2, which happened to be I. Therefore you are left with ipi^3/2 + 2I = -pi^3/4, and I = pi^3/8.

 

[Jason Pham]

I found the mistake!!! Where i evaluated the integral containing (ipi + lnx)^2, i forgot to reconsider the second integral, I2, after proving I1 =-pi^3/2, which happened to be I. Therefore you are left with ipi^3/2 + 2I = -pi^3/4, and I = pi^3/8.

Are you year 12?

 

[quickoats]

Are you year 12?

This is Complex Analysis which is a year 2 uni subject so I’d assume no. You will not get integrals like that in the HSC.

 

[Jason Pham]

This is Complex Analysis which is a year 2 uni subject so I’d assume no. You will not get integrals like that in the HSC.

I see i see, this involves harder integral techniques like complex plane and theorems.