Extension One Revising Game (24 Viewers)

Drongoski · May 5, 2009

what are u doing staying up so late ??

Anyway here's my attempt:

$\100dpi \frac{\left |x+1 \right |}{\left |x+2 \right |}\ <\ 3\ \ \ \Rightarrow \ \ \ \frac{\left |x+1 \right |^{2}}{\left |x+2 \right |^{2}}\ \ <\ \ 3^{2}\ \ \ \ (LHS, \ RHS\ both\ +ve)\\ \\ \\ \therefore \ \ 9\ \ >\ \ \frac{x^{2}+2x+1}{x^{2}+4x+4}\ \ \ \ \ (x \neq -2)\\ \\ \therefore \ \ 9(x^{2}+4x+4)\ >\ x^{2}+2x+1\\ \\ \therefore \ \ 8x^{2}\ +\ 34x\ +\ 35\ \ > \ 0\ \ \Rightarrow \ \ x\ <\ -\frac{5}{2}\ \ \ and\ \ \ x\ >\ -\ \frac{7}{4}$

Hopefully answer correct.

Pwnage101 · May 5, 2009

Drongoski said:
what are u doing staying up so late ??

Anyway here's my attempt:

$\100dpi \frac{\left |x+1 \right |}{\left |x+2 \right |}\ <\ 3\ \ \ \Rightarrow \ \ \ \frac{\left |x+1 \right |^{2}}{\left |x+2 \right |^{2}}\ \ <\ \ 3^{2}\ \ \ \ (LHS. \ RHS\ +ve)\\ \\ \\ \therefore \ \ 9\ \ >\ \ \frac{x^{2}+2x+1}{x^{2}+4x+4}\ \ \ \ \ (x \neq -2)\\ \\ \therefore \ \ 9(x^{2}+4x+4)\ >\ x^{2}+2x+1\\ \\ \therefore \ \ 8x^{2}\ +\ 34x\ +\ 35\ \ > \ 0\ \ \Rightarrow \ \ x\ <\ -\frac{5}{2}\ \ \ and\ \ \ x\ >\ -\ \frac{7}{4}$

Hopefully answer correct.

it might be quiker not to square,. because the the numerator AND the denomenator are both positive, since they are both absoulte values. Then you could take cases.

But yeh your method is fine.

Drongoski · May 5, 2009

Yes there are many ways of skinning this cat. I just don't enjoy doing cases if I can help it. Thank u.

tommykins · May 5, 2009

Yeah i hate cases as well.

Dragonmaster262 · May 5, 2009

Drongoski said:
what are u doing staying up so late ??

Anyway here's my attempt:

$\100dpi \frac{\left |x+1 \right |}{\left |x+2 \right |}\ <\ 3\ \ \ \Rightarrow \ \ \ \frac{\left |x+1 \right |^{2}}{\left |x+2 \right |^{2}}\ \ <\ \ 3^{2}\ \ \ \ (LHS, \ RHS\ both\ +ve)\\ \\ \\ \therefore \ \ 9\ \ >\ \ \frac{x^{2}+2x+1}{x^{2}+4x+4}\ \ \ \ \ (x \neq -2)\\ \\ \therefore \ \ 9(x^{2}+4x+4)\ >\ x^{2}+2x+1\\ \\ \therefore \ \ 8x^{2}\ +\ 34x\ +\ 35\ \ > \ 0\ \ \Rightarrow \ \ x\ <\ -\frac{5}{2}\ \ \ and\ \ \ x\ >\ -\ \frac{7}{4}$

Hopefully answer correct.

I fell asleep early and woke up in the middle of the night.

jet · May 5, 2009

Okay, Parabola, $x^2 = 4ay$
$P \equiv \left [2ap, ap^2 \right ], Q \equiv \left [2aq, aq^2 \right ]$
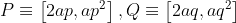
Prove that pq = -1 if the chord PQ passes through (0,a) and hence find the locus of R, the intersection of the normals at P and Q, if the chord passes through the point (0, a). The equation of the chord is $y = \frac{1}{2}(p+q)x - apq$ . You must prove all other formulas. [5 Marks]

gurmies · May 5, 2009

$y = \frac{1}{2}(p+q)x-apq$

$Since \,\, chord \,\, passes \,\, through \,\, focus \,\, (0,a): \,\, a = -apq$

$\therefore pq = -1$

$Now, \,\, x^{2}=4ay$

$y = \frac{x^{2}}{4a}$

$\frac{dy}{dx}=\frac{x}{2a}$

$When \,\, x = 2ap, \,\, M_{T}= \frac{2ap}{2a}=p$

$\therefore M_{N}=-\frac{1}{p}$

$Equation \,\, of \,\, normal \,\, at \,\, (2ap, ap^{2}) \,\, is: y-2ap=-\frac{1}{p}(x-2ap)$

$x + py = 2ap + ap^{3}[1]$

$Similarly \,\, at \,\, Q(2aq, aq^{2}), equation \,\, of \,\, normal \,\, is \,\, x+qy = 2aq + aq^3[2]$

$From \,\, [1], x=2ap + ap^{3}-py$

$Subbing \,\, into \,\, [2]: 2ap + ap^{3}-py+qy=2aq+aq^{3}$

$(q-p)y = 2a(q-p)+a(q-p)(p^{2}+pq+q^{2})$

$y = a(2+p^{2}+pq+q^{2})$

$Subbing \,\, into \,\, [1], x + 2ap +pq^{2}a+ap^{2}q+ap^{3}=2ap + ap^{3}$

$x = -apq(q+p)=a(q+p) \,\, as \,\, pq=-1$

$Back \,\, to \,\, y = a(q^{2}+p^{2}+1) [after \,\, using \,\, pq=-1]$

$y = a[(p+q)^{2}-2pq+1]$

$y = a(p+q)^{2}+3a$

$But \,\, x = a(p+q), \,\, so \,\,(p+q)=\frac{x}{a}$

$\therefore y = a.\frac{x^{2}}{a^{2}}+3a$

$x^{2}=a(y-3a) \,\, is \,\, the \,\, locus \,\, of \,\, R$

NEW QUESTION:

$Prove \,\, that \,\, r\binom{n}{r}=n\binom{n-1}{r-1}. \,\, If \,\, P_{r}=\binom{n}{r}x^{r}(1-x)^{n-r} \,\, find \,\, the \,\, value \,\, of \,\, P_{1}+P_{2}+P_{3}+...+P_{n}. \,\, Also \,\,show \,\, that \,\, P_{1}+2P_{2}+3P_{3}+...+nP_{n}=nx$

azureus88 · May 6, 2009

[maths]r\binom{n}{r}=\frac{rn!}{r!(n-r)!}=n\frac{(n-1)!}{(r-1)!(n-r)!}=n\binom{n-1}{r-1}[/maths]

[maths]P_1+P_2+P_3+...+P_n\\=\sum_{r=1}^{n}\binom{n}{r}x^r(1-x)^{n-r}\\=(1-x+x)^n\\=1[/maths]

[maths]P_1+2P_2+3P_3+...+nP_n\\=\sum_{r=1}^{n}r\binom{n}{r}x^r(1-x)^{n-r}\\=\sum_{r=1}^{n}n\binom{n-1}{r-1}x^r(1-x)^{n-r}\\=nx\sum_{r=1}^{n}\binom{n-1}{r-1}x^{r-1}(1-x)^{n-r}\\=nx(1-x+x)^{n-1}\\=nx[/maths]

New Question:

Differentiate x^x with respect to x.

study-freak · May 6, 2009

azureus88 · May 6, 2009

[maths]\int_{0}^{\pi/4}(\cos x+\sec x)^2dx\\=\int_{0}^{\pi/4}(\cos^2x+sec^2x+2)dx\\=\int_{0}^{\pi/4}(\frac{5}{2}+\frac{1}{2}\cos2x+\sec^2x)dx\\=[\frac{5}{2}x+\frac{1}{4}\sin2x+\tan x]^{\pi/4}_0\\=\frac{5\pi}{8}+\frac{5}{4}[/maths]

New Question:

Find @ if the quadratic y=ax(x-1) is tangent to circle x^2 + y^2 = 1 at x=@.

lolokay · May 7, 2009

azureus88 said:
Find @ if the quadratic y=ax(x-1) is tangent to circle x^2 + y^2 = 1 at x=@.

if you draw the parabola, and the circle, then if they're tangent, the line from the origin to @ must be perpendicular to the tangent (circle geo property).
the gradient of that line is a@(@-1)/@ = a(@-1)
by differentiating we find the gradient of the tangent to be a(2@-1)

so a^2(@-1)(2@-1) = -1 (perpendicular gradients)
a^2 = 1/(1-@)(2@-1)

then, we have
1-@^2 = a^2@^2(1-@)^2
1+@ = @^2(1-@)/(1-@)(2@-1)
= @^2/(2@-1)
(1+@)(2@-1) = @^2
@^2 + @ - 1 = 0
@ = -1/2 +- rt(5)/2
and from the diagram we can see that @ must be positive, so @ = -1/2 + rt5 /2

is there a simpler way to solve this?

AnandDNA · May 7, 2009

Drongoski said:
what are u doing staying up so late ??

Anyway here's my attempt:

$\100dpi \frac{\left |x+1 \right |}{\left |x+2 \right |}\ <\ 3\ \ \ \Rightarrow \ \ \ \frac{\left |x+1 \right |^{2}}{\left |x+2 \right |^{2}}\ \ <\ \ 3^{2}\ \ \ \ (LHS, \ RHS\ both\ +ve)\\ \\ \\ \therefore \ \ 9\ \ >\ \ \frac{x^{2}+2x+1}{x^{2}+4x+4}\ \ \ \ \ (x \neq -2)\\ \\ \therefore \ \ 9(x^{2}+4x+4)\ >\ x^{2}+2x+1\\ \\ \therefore \ \ 8x^{2}\ +\ 34x\ +\ 35\ \ > \ 0\ \ \Rightarrow \ \ x\ <\ -\frac{5}{2}\ \ \ and\ \ \ x\ >\ -\ \frac{7}{4}$

Hopefully answer correct.

my ext teacher sed that we couldnt expand brackets in inequalities

addikaye03 · May 7, 2009

Posting a Q on behalf of lolokay, Question:

For the Geometric series a+ar+ar^2+...
Show that if lim(n-->infinite) Sn=S, then S-Sn=ar^n/1-r

Hence, find the least value of n for which (S-Sn) for the series 6+3+1.5+...is less than 0.0001.

addikaye03 · May 7, 2009

AnandDNA said:
my ext teacher sed that we couldnt expand brackets in inequalities

Remember that|a|=rt(a^2)

Drongoski · May 7, 2009

AnandDNA said:
my ext teacher sed that we couldnt expand brackets in inequalities

Don't understand. Can u plz elaborate.

Dragonmaster262 · May 10, 2009

Find x:

5^2x-5^x-20

study-freak · May 10, 2009

Dragonmaster262 said:
Find x:

5^2x-5^x-20

insufficient information unless you mean 5^2x-5^x-20=0
and I guess you actually mean 5^(2x)-5^x-20=0?

jet · May 10, 2009

$u^2 - u - 20 = 0, $ where $ u = 5^x \text{(i am assuming it is equal to zero)} \\ (u - 5)(u + 4) = 0 \\ u = 5 $ OR $ u = -4 $ which cannot exist as a solution$ \\ \therefore 5^x = 5, x = 1$

There is a question above.

Drongoski · May 10, 2009

I assume question is find x given the expression = 0

5^2x - 5^x - 20 = (5^x)² - 5^x - 20 = 0 (a quadratic eqn in 5^x)

.: (5^x - 5)(5^x + 4) = 0 ==> 5^x = 5 ==> x = 1

5^x + 4 =/= 0

shaon0 · May 10, 2009

addikaye03 said:
Posting a Q on behalf of lolokay, Question:

For the Geometric series a+ar+ar^2+...
Show that if lim(n-->infinite) Sn=S, then S-Sn=ar^n/1-r

Hence, find the least value of n for which (S-Sn) for the series 6+3+1.5+...is less than 0.0001.

S= S {inf} = (a/(1-r))

S-S{n}
= (a/(1-r)) - (a(r^n-1)/r-1)
= (a/(1-r)) - (a(1-r^n)/r-1)
= (ar^n)/(1-r)

S-S{n} < 0.0001
(ar^n/1-r) < 0.0001
(6(1/2)^n/(0.5)) < 0.0001
(1/2)^n < (0.0001/12)
n < ln(0.0001/12)/ln(1/2)
n < 16.87...
Thus, n is 16.

I don't think my answer is right though.

Extension One Revising Game (24 Viewers)

Well-Known Member

Moderator

Well-Known Member

i am number -e^i*pi

Unorthodox top student

Banned

Drover

Member

Bored of

Member

Active Member

Member

The A-Team

The A-Team

Well-Known Member

Unorthodox top student

Bored of

Banned

Well-Known Member

...

Users Who Are Viewing This Thread (Users: 0, Guests: 24)