Re: Year 11 Mathematics 3 Unit Cambridge Question & Answer Thread
 are equal (so they are equal both in length and direction (including ``sense'')).$)
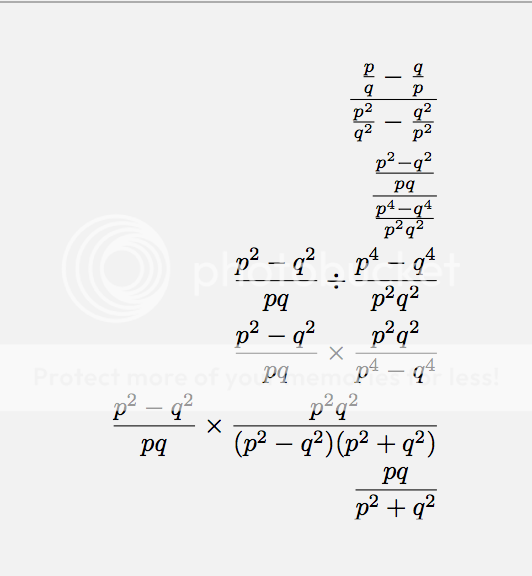
How would you solve:
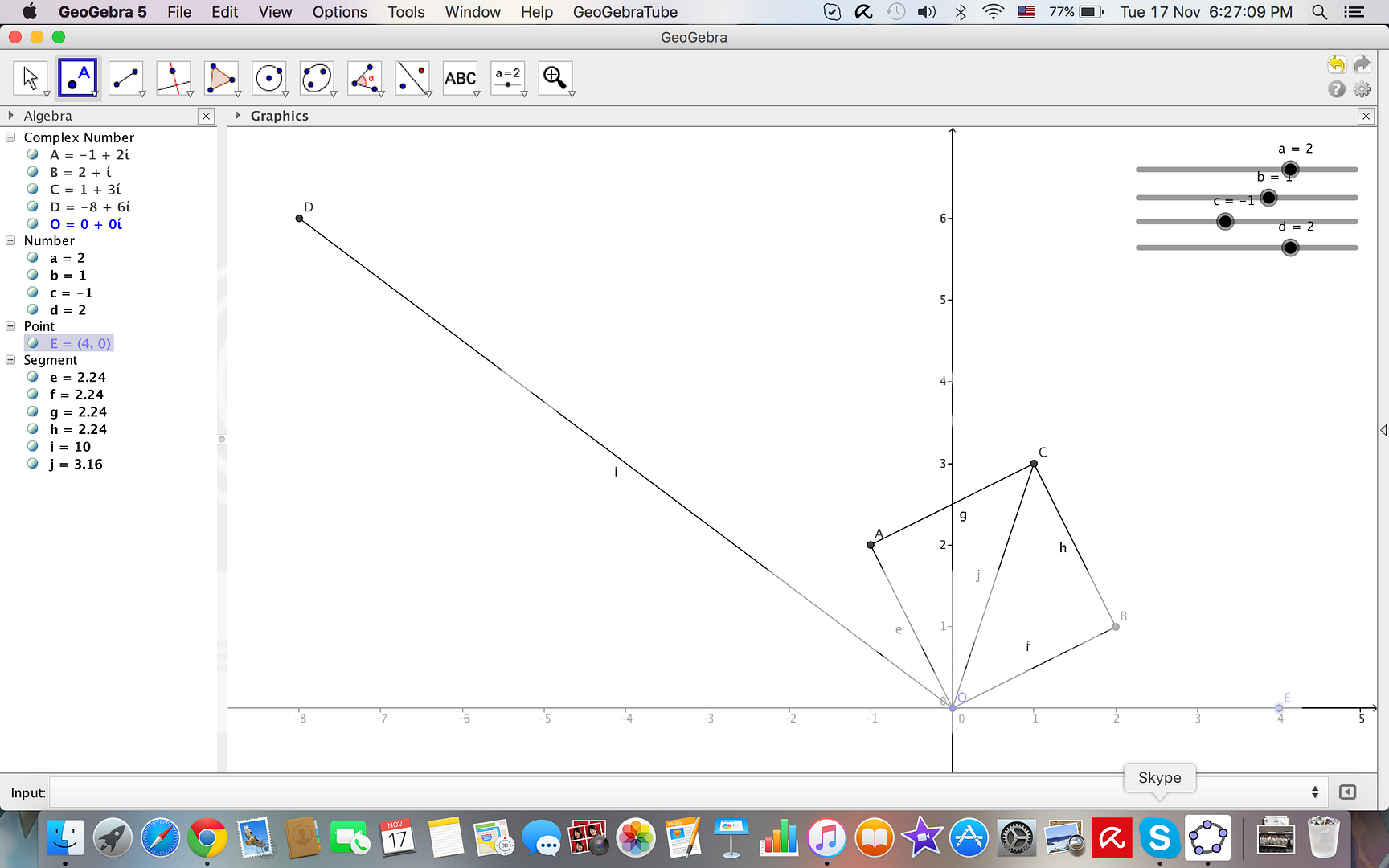
Given that z1 = 1 + i , z2 = 2 + 6i , z3 = -1 + 7i , find the three possible values of z4 so that the points representing z1, z2, z3 and z4 form a parallelogram.