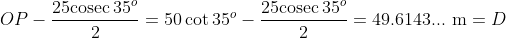motion question (1 Viewer)

cormglakes · Apr 17, 2021

A boat has a speed relative to the water at 3m/s. The boat points 35 degrees to the shore, moving upstream on a river flowing 0.75m/s. How much time does it take for the boat to reach the opposite shore if it the river is 50m wide?

CM_Tutor · Apr 19, 2021

cormglakes said:
A boat has a speed relative to the water at 3m/s. The boat points 35 degrees to the shore, moving upstream on a river flowing 0.75m/s. How much time does it take for the boat to reach the opposite shore if it the river is 50m wide?

Sounds like a vectors question in the MX1 syllabus, so I will offer first the solution that applies vectors. If this is meant as a question at the Advanced Maths level, a different method (described below) is needed.

MX1 Approach

Let's assume that the two banks of the river are straight lines and are parallel, so that there is fixed perpendicular distance of 50 m between them.
Take the origin at the boat's starting position with the river bank being the $x$ -axis so that the far bank is located along the line $y=50$ .

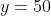
Now, take the river as flowing from right to left, so that the positive $x$ -direction corresponds to upstream. It follows that, since the water is flowing in the negative direction at a speed of 0.75 m s^-1, the vector corresponding to the flow of the river is $-0.75\overrightarrow{i}$ .

The boat is travelling in a direction that is inclined at and angle of 35^o from the shore and in the upstream direction. The unit vector for this direction is

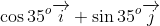
$\cos{35^o}\overrightarrow{i} + \sin{35^o}\overrightarrow{j}$

Since the boat is moving at 3 m s^-1 relative to the water, the boat's velocity relative to the water is a vector along the given direction and with magnitude of 3. That is, it is

$3\left(\cos{35^o}\overrightarrow{i} + \sin{35^o}\overrightarrow{j}\right)$

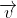
Let the velocity of the boat across the river (relative to the bank) be $\overrightarrow{v}$ , which is made up of the motion of the boat relative to the water and the motion of the water itself. Consequently,

$\begin{align*} \overrightarrow{v} &= 3\left(\cos{35^o}\overrightarrow{i} + \sin{35^o}\overrightarrow{j}\right) + -0.75\overrightarrow{i} \\ &= \left(3\cos{35^o} - \frac{3}{4}\right)\overrightarrow{i} + 3\sin{35^o}\overrightarrow{j} \\ &= \frac{3}{4}\left(4\cos{35^o} - 1\right)\overrightarrow{i} + 3\sin{35^o}\overrightarrow{j} \end{align*}$

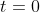
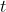
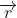
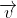
Taking the origin of time ( $t=0$ ) as the time when the boat departs, then the position vector of the boat at time $t$ , $\overrightarrow{r}$ , is found by integrating $\overrightarrow{v}$ with respect to $t$ :

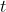
$\overrightarrow{r} = \frac{3t}{4}\left(4\cos{35^o} - 1\right)\overrightarrow{i} + 3t\sin{35^o}\overrightarrow{j}$

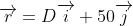
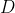
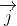
The boat reaches the far bank when $\overrightarrow{r} = D\overrightarrow{i} + 50\overrightarrow{j}$ , where $D$ is the distance that the boat has travelled along the bank relative to its starting position. Equating the $\overrightarrow{j}$ components allows the time taken to cross the river to be determined:

$\begin{align*} 3t\sin{35^o} &= 50 \\ t &= \frac{50}{3\sin{35^o}} \\ &= 29.05744... \text{ s} \\ &\approx 29.1 \text{ s (1 dec. pl.)} \end{align*}$

----

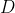
Now, it was not requested, but we can use the above result to find the distance $D$ as it also occurs at the time when the boat reaches the far bank:

$\begin{align*} D &= \frac{3}{4}(4\cos{35^o} - 1) \times \frac{50}{3\sin{35^o}} \\ &= \frac{4\cos{35^o} - 1}{2} \times \frac{25}{\sin{35^o}} \\ &= \frac{25(4\cos{35^o} - 1)}{2\sin{35^o}} \\ &= 49.614315... \text{ m} \\ &\approx 49.6 \text{ m (1 dec. pl.)} \end{align*}$

So, the boat takes 29.1 s to cross the river, and lands at a position 49.6 m upstream from the point on the far bank opposite its starting point.

----

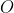
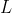
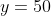
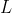
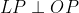
We can do a check to estimate the solution by neglecting the flow of the river. If the river was not flowing, the path would be a straight line with $O$ being the origin, $L$ being the point on the other bank (ie. on the line $y = 50$ ) where the boat lands, and $P$ being the point on the starting bank which is directly opposite $L$ - in other words, where $LP \perp OP$ . 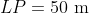From the given information, $\angle{LOP} = 35^o$ , $LP = 50 \text{ m}$ , and so

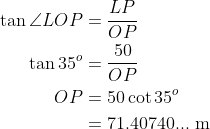
$\begin{align*} \tan{\angle{LOP}} &= \frac{LP}{OP} \\ \tan{35^o} &= \frac{50}{OP} \\ OP &= 50\cot{35^o} \\ &= 71.40740... \text{ m} \end{align*}$

The river flow is forcing the boat downstream, so we know it will actually land downstream of this point 71.4 m upstream, which is consistent with the above calculation showing an arrival position of 49.6 m along the opposite bank in the upstream direction.

----

Advanced Maths rather than MX1 approach

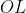
The above triangle trigonometry allows the distance $OL$ to be calculated, either using Pythagoras' Theorem

$\begin{align*} OL^2 &= OP^2 + PL^2 \\ &= 50^2\cot^2{35^o} + 50^2 \\ &= 50^2\left(\cot^2{35^o} + 1\right) \\ &= 50^2\text{cosec}^2{35^o} \\ OL &= 50\,\text{cosec}\,35^o \qquad \qquad \text{as $OL > 0$} \end{align*}$

or by trigonometry

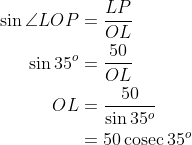
$\begin{align*} \sin{\angle{LOP}} &= \frac{LP}{OL} \\ \sin{35^o} &= \frac{50}{OL} \\ OL &= \frac{50}{\sin{35^o}} \\ &= 50\,\text{cosec}\,35^o \end{align*}$

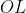
And the boat travels along $OL$ at 3 m s^-1, and so the time taken will be

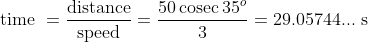
$\text{time } = \frac{\text{distance}}{\text{speed}} = \frac{50\,\text{cosec}\,35^o}{3} = 29.05744... \text{ s}$

Now, the boat will be pushed downstream by the river flow, which will alter the path and the landing position. However, the motion can be considered as having a component along the river and a perpendicular motion across it. The river flow will alter the former but not the latter, and it follows that the time taken for the crossing is the same. The only effect of the river flow is to change the landing position by a distance of

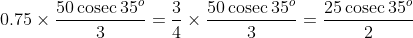
$0.75 \times \frac{50\,\text{cosec}\,35^o}{3} = \frac{3}{4} \times \frac{50\,\text{cosec}\,35^o}{3} = \frac{25\,\text{cosec}\,35^o}{2}$

Consequently, the landing position will be

$OP - \frac{25\text{cosec}\,35^o}{2} = 50\cot{35^o} - \frac{25\text{cosec}\,35^o}{2} = 49.6143... \text{ m}= D$

CM_Tutor · Apr 20, 2021

Extensions... An MX1 paper could also ask that you prove that the path taken by the boat from $O$ to its landing point is a straight line, or for other details:

It has already been established that the position vector for the boat, relative to its starting position at $O$ , at time $t$ is given by

$\overrightarrow{r} = \frac{3t}{4}\left(4\cos{35^o} - 1\right)\overrightarrow{i} + 3t\sin{35^o}\overrightarrow{j}$

from which we know that the boat's position at time $t$ is

$(x, y) = \left(\frac{3t}{4}\left(4\cos{35^o} - 1\right), 3t\sin{35^o}\right)$

and the equation of the path can be found by treating $t$ as a parameter and eliminating it to form the path equation in $x$ and $y$ .

$\begin{align*} \text{We have} \qquad y &= 3t\sin{35^o} \\ \text{Rearranging, this becomes} \qquad t &= \frac{y}{3\sin{35^o}} \\ \text{Substituting into the equation for $x$} \qquad x &= \frac{3\left(4\cos{35^o} - 1\right)}{4} \times \frac{y}{3\sin{35^o}} \\ y &= \frac{4 \times 3x\sin{35^o}}{3\left(4\cos{35^o} - 1\right)} \\ &= \frac{4x\sin{35^o}}{4\cos{35^o} - 1} \end{align*}$

This is clearly a straight line through the origin as it takes the form $y = mx$ where $m$ is a real constant. Thus, the path along which the boat travels is a straight line. This path equation can be used to confirm the value of $D$ as we know that the point $(D, 50)$ lies on the line:

$\begin{align*} \text{Putting $(D, 50)$ into the equation:} \qquad 50 &= \frac{4D\sin{35^o}}{4\cos{35^o} - 1} \\ D &= \frac{50\left(4\cos{35^o} - 1\right)}{4\sin{35^o}} \\ &= \frac{25\left(4\cos{35^o} - 1\right)}{2\sin{35^o}} \qquad \qquad \text{as expected} \end{align*}$

Since the path is a line, the distance travelled by the boat from $(0, 0)$ to $(D, 50)$ is $\sqrt{D^2 + 50^2}$ using the distance formula (or Pythagoras' Theorem) and since the time taken to cross the river is known, the speed of the boat (relative to the shore) is

$\begin{align*} \text{speed } &= \frac{\text{distance}}{\text{time}} \\ &= \sqrt{D^2 + 50^2} \times \frac{3\sin{35^o}}{50} \\ &= \frac{3\sin{35^o}}{50} \sqrt{\left(\frac{25\left(4\cos{35^o} - 1\right)}{2\sin{35^o}}\right)^2 + 50^2} \\ &= 3 \sqrt{\frac{\sin^2{35^o}}{50^2}\left(\frac{25^2\left(4\cos{35^o} - 1\right)^2 + 50^2 \times 4\sin^2{35^o}}{4\sin^2{35^o}}\right)} \\ \\ &= 3 \sqrt{\frac{\sin^2{35^o}}{2^2 \times 25^2}\left(\frac{25^2\left(4\cos{35^o} - 1\right)^2 + 2^2 \times 25^2 \times 4\sin^2{35^o}}{4\sin^2{35^o}}\right)} \\ &= 3 \sqrt{\frac{1}{2^2}\left(\frac{\left(4\cos{35^o} - 1\right)^2 + 16\sin^2{35^o}}{4}\right)} \\ &= 3 \sqrt{\frac{16\cos^2{35^o} - 8\cos{35^o} + 1 + 16\sin^2{35^o}}{4^2}} \\ &= \frac{3}{4} \sqrt{16 - 8\cos{35^o} + 1} \qquad \text{as $\sin^2{35^o} + \cos^2{35^o} = 1$} \\ &= \frac{3}{4} \sqrt{17 - 8\cos{35^o}} \text{ m s}^{-1} \\ &\approx 2.42111... \text{ m s}^{-1} \end{align*}$

You can easily check to confirm that this average speed is, in fact, $\left|\overrightarrow{v}\right|$ .

motion question (1 Viewer)

cormglakes

New Member

CM_Tutor

Moderator

CM_Tutor

Moderator

Users Who Are Viewing This Thread (Users: 0, Guests: 1)