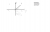Trig question 2 unit prelim (1 Viewer)

[Sy123]

In the preliminary version, its called ASTC, (All Sine Tan Cos)
Here is a crappy diagram

The logic behind ASTC is from trigonometric graphs, when you graph y=sin(x), between 270 and 360 degrees (3pi/2 and 2pi) the curve of y=sinx is below the x-axis, therefore it is negative, and since the graph is symmetrical, you get the exact answer.

In this diagram, the higlighted part, is where Sin is negative.


And the logic behind sin(90-x) = cosx




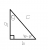
Take a normal right angle triangle, one angle is theta, the other is 90 - theta (angle sum of triangle)
In here I put x as theta, because Im adumb with latex
According to the triangle:

sin(x) = b/a

but if you take the cosine of the OTHER angle

cos(90-x) = b/a

Therefore:

cos(90-x)=sin(x)

and vice versa

 

[zeebobDD]

you should really try and go through the fundamentals of the unit circle before you attempt the questions

 

[barbernator]

IMO Here is the easiest way to understand how these two functions are simplified.

tan(90-x)=cot(-x). Think of a right angled triangle. For whichever value x is defined as, the other acute angle will be 90-x. Therefore, the tan of one angle (Opposite/adjacent) is obviously equal to (adjacent/opposite) from the other angles perspective. as cot is the reciprocal function of tan, it is (adjacent/opposite), and from the right angled triangle definition, hence tan(90-x)=cot(-x) obviously is true.

sin(360-x)=sin(-x)=-sin(x). As these are defined within a revolution of 360 degrees, the same value for sin(x) occurs after every revolution of 360 degrees. This shows the first part of our equation sin(360-x)=sin(-x). Now by the quadrants, for sin functions, the right quadrants are positive definite, and the left quadrants are negative definite. but for each addition of 90 degrees to an angle, the absolute value of the sine function doesnt change. Therefore we have an odd function. and hence sin(-x)=-sin(x).

 

[Sanjeet]

For those of you still wondering about sin.


You're welcome

 

[Carrotsticks]

For those of you still wondering about sin.


You're welcome

Alternatively, you could sketch y=sin(x), then y=cos(x-90), then observe they are the same curve ie: