Re: HSC 2015 4U Marathon
Consider a small slice of the volume desired. Approximate this volume by taking one of the cross-sectional planes of the volume at some value of

, and extending the section out by some value of

, which we shall call
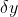
. Then this volume will approximate a small slice of the volume.
The base of this has a length that is dependent upon the x-co-ordinates corresponding with the value of

we chose. Solving for

, we get
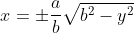
.
Because we want the length of the base, we take the positive value and double it. Then the length of the base is simply

The shape of the cross-sectional slice is similar to the shape from a). Scaling down this shape allows us to find the area of the cross section. We express the area of the slice as a percentage of the original shape in a). This percentage is found by dividing the cross-sectional base value by the base value of the original shape. So the percentage is

The area of the slice is then the percentage times the original area, i.e.
}\right ) \cdot \frac{1}{b}\sqrt{b^2-y^2}=a\left(\sqrt{2}+\ln{(1+\sqrt{2})}\right )\sqrt{b^2-y^2})
We now have the area of the slice, and hence, the volume of the slice is
}\right )\sqrt{b^2-y^2}\delta y)
Summing this from
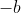
to

will approximate the entire volume. However, observe that the solid is symmetrical along the x-axis. This means we only have to double the volume of half the solid, i.e, we can sum from

to

instead.
} \right )\sqrt{b^2-y^2}\delta y = 2a\left(\sqrt{2}+\ln{(1+\sqrt{2})} \right )\sum_{y=0}^{b}\sqrt{b^2-y^2}\delta y)
since
} \right ))
is constant.
As we make
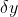
smaller and smaller, the volume approximates the true volume of the solid. In the limit, it becomes equal to the true volume (Rigorous justification beyond High School level

).
i.e. ignoring the outside constant for now, we have

This integral can now be evaluated to obtain the volume, which matches the given answer exactly.


